
Bézout's identity suggests that numerators exist such that the sum of these fractions equals the original rational function. It involves factoring the denominators of rational functions and then generating a sum of fractions whose denominators are the factors of the original denominator.

What is partial fraction decomposition? Partial fraction decomposition is a useful process when taking antiderivatives of many rational functions. Get immediate feedback and guidance with step-by-step solutions
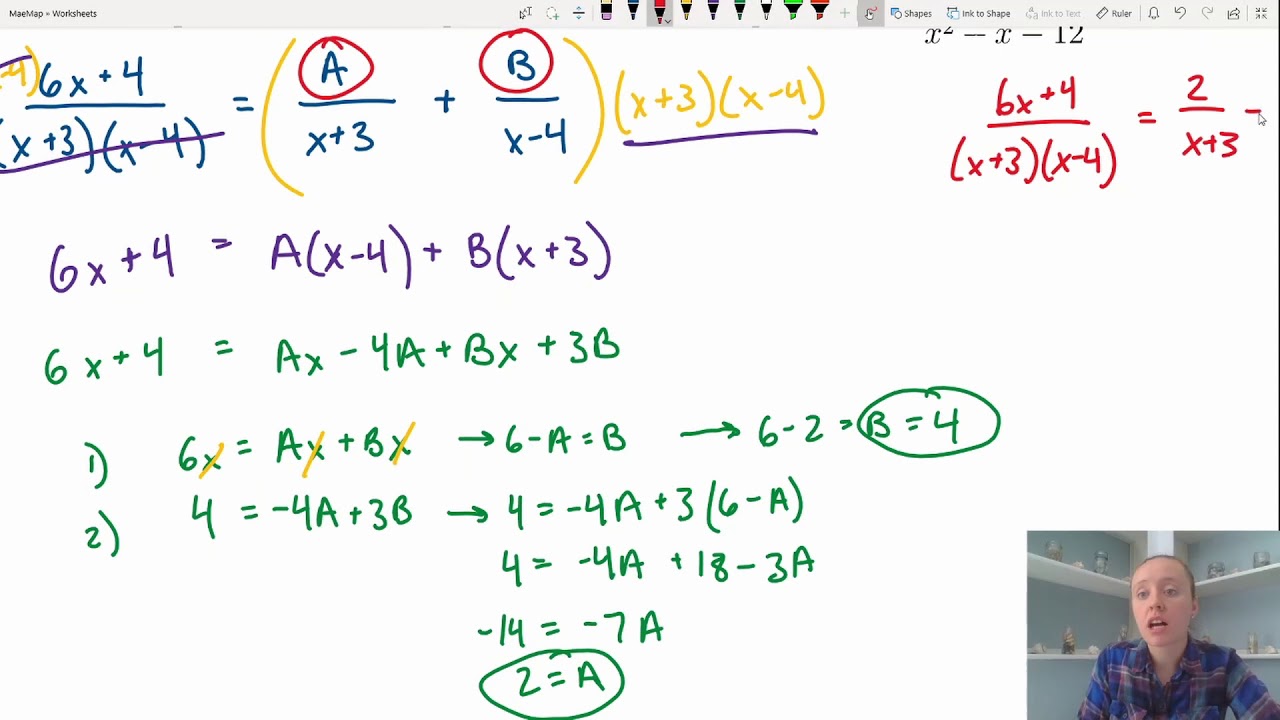
find partial fractions for 5/((x + 1)(x^2 - 7)).partial fraction decomposition x^2/(x^2 + 7x + 10).
Partial fractions calculator with steps how to#
Here are some examples illustrating how to ask about applying partial fraction decomposition. To avoid ambiguous queries, make sure to use parentheses where necessary. It can also utilize this process while determining asymptotes and evaluating integrals, and in many other contexts including control theory.Įnter your queries using plain English. Given any rational function, it can compute an equivalent sum of fractions whose denominators are irreducible. Wolfram|Alpha provides broad functionality for partial fraction decomposition.

"Fast Algorithms for Partial Fraction Decomposition". "Recursive formulas for the partial fraction expansion of a rational function with multiple poles". "An algorithm for the incomplete decomposition of a rational function into partial fractions". "Recursive techniques for obtaining the partial fraction expansion of a rational function". " Algorithms for partial fraction decomposition and rational function integration." Proceedings of the second ACM symposium on Symbolic and algebraic manipulation. It can also be found with limits (see Example 5). This solution can be found using any of the standard methods of linear algebra. In this way, a system of linear equations is obtained which always has a unique solution. Since two polynomials are equal if and only if their corresponding coefficients are equal, we can equate the coefficients of like terms. We then obtain an equation of polynomials whose left-hand side is simply p( x) and whose right-hand side has coefficients which are linear expressions of the constants A ir, B ir, and C ir. The most straightforward method is to multiply through by the common denominator q( x). There are a number of ways the constants can be found. Here, P( x) is a (possibly zero) polynomial, and the A ir, B ir, and C ir are real constants. In symbols, the partial fraction decomposition of a rational fraction of the form f ( x ) g ( x ), The concept was discovered independently in 1702 by both Johann Bernoulli and Gottfried Leibniz.
Partial fractions calculator with steps series#
The importance of the partial fraction decomposition lies in the fact that it provides algorithms for various computations with rational functions, including the explicit computation of antiderivatives, Taylor series expansions, inverse Z-transforms, and inverse Laplace transforms. In algebra, the partial fraction decomposition or partial fraction expansion of a rational fraction (that is, a fraction such that the numerator and the denominator are both polynomials) is an operation that consists of expressing the fraction as a sum of a polynomial (possibly zero) and one or several fractions with a simpler denominator. ( September 2012) ( Learn how and when to remove this template message) Please help to improve this article by introducing more precise citations.
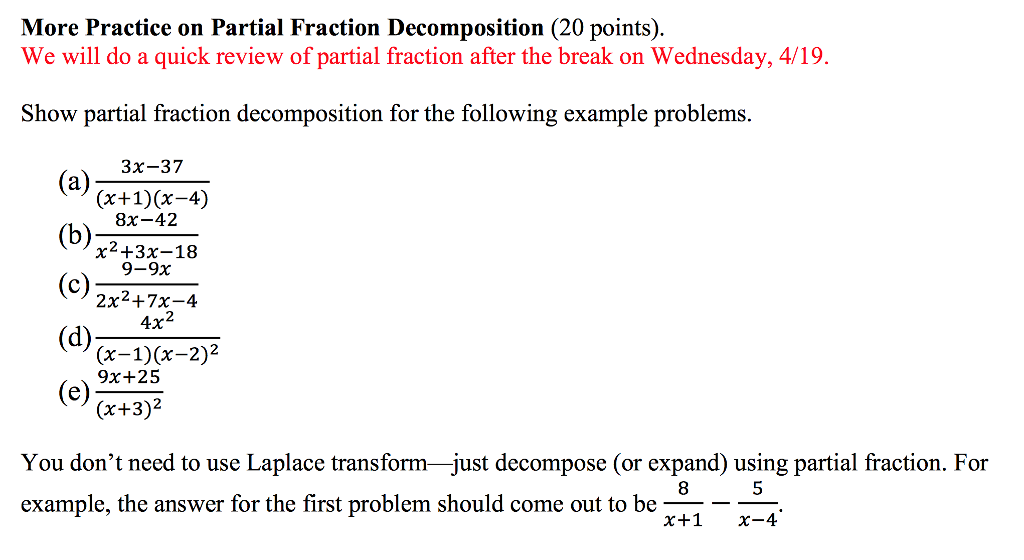
This article includes a list of general references, but it lacks sufficient corresponding inline citations.


 0 kommentar(er)
0 kommentar(er)
